Hahn embedding theorem
In mathematics, especially in the area of abstract algebra dealing with ordered structures on abelian groups, the Hahn embedding theorem gives a simple description of all linearly ordered abelian groups.
The theorem states: Any linearly ordered abelian group  can be embedded as an ordered subgroup of the additive group ℝΩ endowed with a lexicographical order, where ℝ is the additive group of real numbers (with its standard order), and Ω is the set of Archimedean equivalence classes of
can be embedded as an ordered subgroup of the additive group ℝΩ endowed with a lexicographical order, where ℝ is the additive group of real numbers (with its standard order), and Ω is the set of Archimedean equivalence classes of  .
.
Let 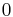 denote the identity element of
denote the identity element of  . For any nonzero
. For any nonzero 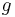 ∈
∈ , exactly one of the elements
, exactly one of the elements 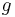 or
or 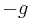 is greater than
is greater than 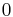 ; denote this element by
; denote this element by 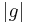 . Two nonzero elements
. Two nonzero elements  ∈
∈ are Archimedean equivalent if there exist natural numbers
are Archimedean equivalent if there exist natural numbers 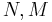 ∈ℕ such that
∈ℕ such that 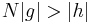 and
and 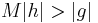 . (Heuristically: neither
. (Heuristically: neither 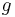 nor
nor 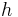 is "infinitesimal" with respect to the other). The group
is "infinitesimal" with respect to the other). The group  is Archimedean if all nonzero elements are Archimedean-equivalent. In this case, Ω is a singleton, so ℝΩ is just the group of real numbers. Then Hahn's Embedding Theorem reduces to Hölder's theorem (which states that a linearly ordered abelian group is Archimedean if and only if it is a subgroup of the ordered additive group of the real numbers).
is Archimedean if all nonzero elements are Archimedean-equivalent. In this case, Ω is a singleton, so ℝΩ is just the group of real numbers. Then Hahn's Embedding Theorem reduces to Hölder's theorem (which states that a linearly ordered abelian group is Archimedean if and only if it is a subgroup of the ordered additive group of the real numbers).
(Gravett 1956) gives a clear statement and proof of the theorem. The papers of (Clifford 1954) and (Hausner & Wendel 1952) together provide another proof. See also (Fuchs & Salce 2001, p. 62).
References
- Fuchs, László; Salce, Luigi (2001), Modules over non-Noetherian domains, Mathematical Surveys and Monographs, 84, Providence, R.I.: American Mathematical Society, ISBN 978-0-8218-1963-0, MR1794715
- Hahn, H. (1907), "Über die nichtarchimedischen Größensysteme." (in German), Sitzungsberichte der Kaiserlichen Akademie der Wissenschaften, Wien, Mathematisch - Naturwissenschaftliche Klasse (Wien. Ber.) 116: 601–655
- Gravett, K. A. H. (1956), "Ordered Abelian Groups", Quarterly Journal of Mathematics of Oxford Series 2 7: 57–63, doi:10.1093/qmath/7.1.57
- Clifford, A.H. (1954), "Note on Hahn's Theorem on Ordered Abelian Groups", Proceedings of the American Mathematical Society 5 (6): 860–863
- Hausner, M.; Wendel, J.G. (1952), "Ordered vector spaces", Proceedings of the American Mathematical Society 3: 977–982, doi:10.1090/S0002-9939-1952-0052045-1